HEAT TRANSFER COEFFICIENT
Heat transfer coefficient is a quantitative characteristic of convective heat transfer between a fluid medium (a fluid) and the surface (wall) flowed over by the fluid. This characteristic appears as a proportionality factor a in the Newton-Richmann relation
where 
is the heat flux density on the wall, Tw the wall temperature, Tt the characteristic fluid temperature, e.g., the temperature Te far from the wall in an external flow, the bulk flow temperature Tb in tubes, etc. The unit of measurement in the international system of units (SI) (see International system of units) is W/(m2K), 1 W/(m2K) = 0.86 kcal/(m2h°C) = 0.1761 Btu/(hft2°F) or 1 kcal/(m2h°C) = 1.1630 W/(m2K), 1 Btu/(hft2°F) = 5.6785 W/(m2K). The heat transfer coefficient has gained currency in calculations of convective heat transfer and in solving problems of external heat exchange between a heat conducting solid medium and its surroundings. Heat transfer coefficient depends on both the thermal properties of a medium, the hydrodynamic characteristics of its flow, and the hydrodynamic and thermal boundary conditions. Using the methods of similarity theory, the dependence of heat transfer coefficient on many factors can be represented in many cases of practical importance as compact relations between dimensionless parameters, known as similarity criteria. These relations are said to be generalized or similarity equations (formulas). The Nusselt number Nu = αl/λf or the Stanton number St = 
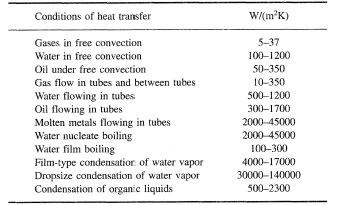
is used as a dimensionless number for heat transfer in these equations, where 1 is the characteristic dimension of the surface in the flow, 
the mass velocity of the fluid flow, λf and Cpf the fluid thermal conductivity and heat capacity. When solving the problems of heat conduction in a solid, the distribution of heat transfer coefficient α between the body and its surroundings is often given as a boundary condition. Here, it is useful to use a dimensionless independent parameter, the Biot number Bi = αl/λs , where λs is the thermal conductivity of a solid and 1 its characteristic dimension. The dependence of the Nu and St numbers on the Re and Pr numbers plays an essential role in heat transfer by forced convection. In the case of fully developed heat transfer in a circular tube with laminar fluid flow the Nusselt number is a constant, namely Nu = 3.66 at a constant wall temperature and 4.36 at a constant heat flux (see Tubes (single-phase heat transfer in)). In the case of free convection, the Nu number depends on the Gr and Pr numbers. When the heat capacity of the fluid varies substantially, the heat transfer coefficient is frequently determined in terms of enthalpy difference (hw – hf). The concept of heat transfer coefficient is also used in heat transfer with phase transformations in liquid (boiling, condensation). In this case the liquid temperature is characterized by the saturation temperature Ts. The order of magnitude of heat transfer coefficient for different cases of heat transfer is presented in Table 1.
When analyzing internal heat transfer in porous bodies, i.e., convective heat transfer between a rigid matrix and a fluid permeating through it, use is often made of the volumetric heat transfer coefficient
where qv is the heat flux passing from the rigid matrix to the fluid in a unit volume of a porous body, Tw the local temperature of the matrix, and Tf the local bulk temperature of the fluid.
It should be emphasized that the constancy of α over a wide range of 
and ΔT (other conditions being equal) is encountered only in the case of convective heat transfer when the physical properties of fluid change only slightly during heat transfer. Under convective heat transfer in a fluid with varying properties and in boiling, heat transfer coefficient may substantially depend on 
and ΔT . In these cases an increase of heat flux may give rise to hazardous phenomena such as burnout (transition heat flux) and deterioration of turbulent heat transfer in tubes. If the 
(ΔT ) is nonlinear, it appears inappropriate to represent it in terms of the coefficient α when analyzing, for example, boiling stability.
An overall heat transfer coefficient
where Tf1 and Tf2 are the temperatures of the heating and heated liquids, is used in calculations of heat transfer between two fluids through the separating wall. The U values for the most commonly used wall configurations are determined by the formulas
for a plane multilayer wall,
for a cylindrical multilayer wall, and
for a spherical multilayer wall.
Here D1 and D2 are the internal and external diameters of the wall, D the reference diameter by which a reference heat transfer surface is determined, Si, Di, Di+1 and λi are the thickness, internal and external diameters, and the thermal conductivity of the ith layer. The first and the third terms in brackets are said to be thermal resistances of heat transfer. In order to lower them the walls are finned and various methods of heat transfer augmentation are used. The second term in brackets is said to be the thermal resistance of the wall, which may greatly increase as a result of wall contamination, such as scale and ash build-up, or poor heat transfer between the wall layers. The values of α and U for a small element of heat transfer surface are called local ones. If they do not vary greatly then, in practical calculations of heat transfer on finite-size surfaces, we use the mean values of the coefficients and the heat transfer equation
where A is the reference heat transfer surface, and 
(ofen mean logarithmic 
) temperature drop (see Mean Temperature Difference).
Table 1. Approximate values of heat transfer coefficient
See more : https://mptmeco.com/san-pham/thiet-bi-trao-doi-nhiet-calorifer.html
